Let
us define the following variables:
Thus, we
can use the above definitions to describe the total moles in a batch system:
Let us
assume a reaction between reactants A and B to produce products C and D
occurring in a constant volume batch reactor. With A as the limiting reactant
and the basis of the reaction:
A table used to compute the changes and remaining quantities of each substance in the reaction in a constant volume batch reactor can be developed as follows:
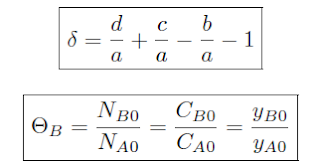
Using the mole fraction definition of Θ, for a constant volume batch reactor:
This
formula is for an arbitrary species i ≠ A, Species A is the limiting
reagent and i in the numerator represents the stoichiometric coefficient
of species i. For the ± , the addition represents generation of products
and the subtraction is for the consumption of reactants.
The
stoichiometric coefficient is defined as follows where for a substance I
with stoichiometric number i. It is positive for products and negative
for reactants:
In a
gas-phase reaction, the constant-volume condition tends to exist when n moles
of reactant form n moles of product and when there is no change in temperature
or pressure (i.e., ideal gas law states that volume is unchanged).
In liquid-phase
reaction, the solvent dominates the solution, hence the density of the solute
insignificantly impacts the system hence making most liquid-phase reactions
essentially constant-volume.