Entropy generation calculations inform us about the inefficiency of a process, i.e., the higher the generation of entropy, the higher the amount of lost work.
In
some cases, we can set entropy generation to zero and use the first law of
thermodynamics to assess the best-case scenario from a given process.
Furthermore, entropy generation calculation tells us the
feasibility of a proposed process by satisfying ΔSuniverse =
Sgen > 0.
For the following examples, Appendix B for steam values that I have referred to in these questions was obtained from:
M.D Koretsky, Engineering and Chemical Thermodynamics, Wiley, 2004.
Question 1
Two
moles of an ideal gas in a piston-cylinder system at 150°C are compressed
isothermally but irreversibly from 2bar to 8bar. The actual work required for
compression is 30% greater than the reversible work for the same compression.
If
the heat removed from the gas is discarded to a heat reservoir at 25°C,
calculate the entropy change of the gas and the heat reservoir.
Solution
System: Gas contained in the piston-cylinder.
NOTE: It is possible to calculate the entropy change of the system as follows:
Question 2
The following diagram illustrates a proposed process. Determine whether it is a feasible process.
Solution
Question 3
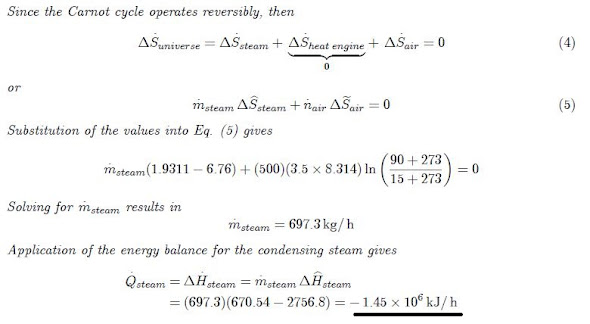
500 kmol/h of an air stream at a pressure of 2 atm is
to be heated from 15°C to 90°C in a heat exchanger with 600 kPa steam
condensing in the shell side of the heat exchanger. Steam enters the heat
exchanger as saturated vapor and exits as saturated liquid.
a) How much steam will be needed if a conventional heat exchanger is used? What is the total entropy change during this process?
b) How much steam is needed if the process of heating the air was carried out by using the heat transferred from the steam to drive a reversible Carnot engine and by using the air stream as the heat sink? What would be the total work output of the engine?
Solution
a) System: Heat Exchanger
b)












No comments:
Post a Comment